The Shape of Music | The Circle of Fifths Part 1

Written by Mete Bakircioglu; Edited by Jackson Poling

Welcome back to “The Shape of Music,” a five-part series where we take you through the magic of music—how it works and what makes it sound so good. So far, we’ve learned about rhythm (the heartbeat of music) and melody (the “meat and potatoes” of music). We explored the musical alphabet through studying the keyboard. Finally, we navigated the musical language: how different families of instruments produce different colors of sounds. This is the first part of a two-part lesson where we dissect and analyze the circle of fifths, the treasure map to music theory.

Listen to the podcast:

Pythagoras:

Consider how you draw a star: how many points does it have? Now take a look at your hand: how many fingers do you see? Bonus points if you can get this one: how many senses do we have? The answer to all of these questions is five. The number five holds a really special place in nature, and by extension, in music. Notice how this is the fifth episode of “The Shape of Music?” It’s not a coincidence…

The circle of fifths is a diagram (just like the diagrams of the water cycle and parts of a flower you see in school) of the 12 unique tones we have in western music. Let’s back up about 2600 years ago in Ancient Greece. A philosopher by the name of Pythagoras, creator of the first circle of fifths, was proving all sorts of wild mathematical and scientific theories. But why is a mathematician coming up with diagrams for music theory? It’s because music theory is rooted in math, and as we’ve been learning, there’s a lot of overlap between the two subjects.

Here’s what Pythagoras discovered: if you take any pitch and multiply its frequency by the fraction 3/2, you find the perfect fifth above that pitch. Take the A above middle C for example. If you’ve ever heard an orchestra warm up before a performance, you’ll recognize that this is the note they use to tune themselves: an A with a frequency of 440 megahertz. If you have a calculator handy, or can do the math, you’ll find that 440 times 3/2 is 660 megahertz. So what tone is this? Let’s look back to our circle. The fifth note in the A major scale is E. That means this E has a frequency of 660 megahertz. And then if you take the B above that, you multiply again by 3 over 2 for the frequency and get roughly 990 megahertz. The reason I say “roughly” is because it’s actually 987.77 megahertz.

Key Signatures:

Now let’s talk about key signatures. A key signature is how many sharps (♯) or flats (♭) are in a key. The way I like to think of this is by imagining my own signature. Each of our signatures is unique to us; it’s the same with a key’s signature. You can tell the number of sharps in a key by counting what position it is clockwise on the clock, starting from C. A, for example, is at the 3 o’clock position, meaning it has three sharps. When you’re trying to find the sharps for any key, start on F at 11 o’clock and go clockwise for as many positions the tone is to the right of C. So for A, the three sharps are F, C, and G. How many sharps does the key of B have, and which ones are they?

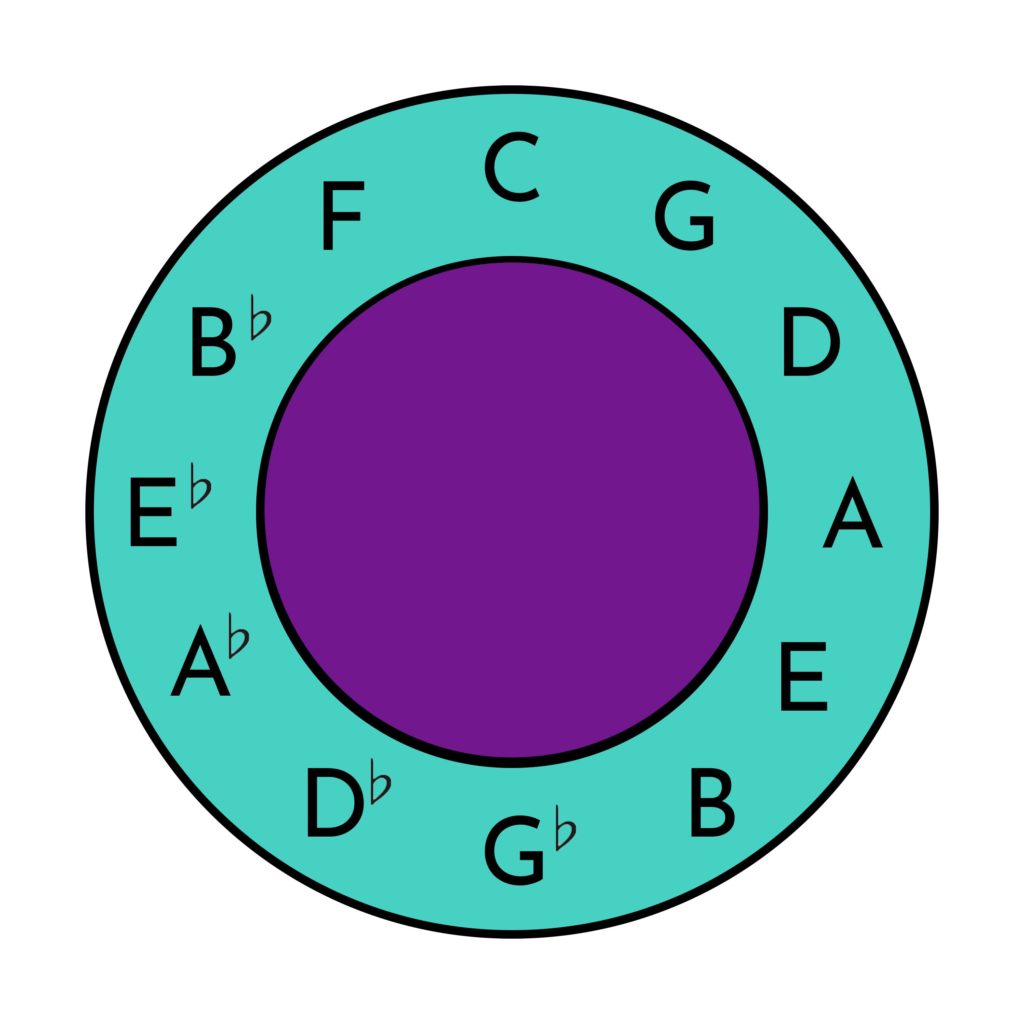
To figure out the key signature for flat keys, the number of flats is how many positions the key is to the left of C. So for E flat, we are one, two, three keys to the left of C, which means there are three flats. So which notes are flat? To figure this out, we’re starting not at the 11 o’clock position at F, but at the 5 o’clock position at B. Since E flat is three positions counter clockwise from C, we start at B and go up two more. So there’s B flat, up one from that is E flat, then A flat. So how about the key of F? Well since F is only one key to the left of C, we know that B flat is the only flat.

Bright and Dark Sides of the Moon:

Another neat thing to note about the sides of the circle is that the right side is usually associated with brighter sounds while the left side is associated with darker sounds (audio clips of both featured below). I learned about this by imagining the circle of fifths as the moon. Since the sun only shines on one side of the moon, the other side is always dark. So imagine that the right side of the circle is the bright side of the moon and the left half of the circle is the dark side of the moon. This explains more about the color of music which we explored last episode, and how musicians can choose a key to fit the mood they’re after.

Using the Circle of Fifths:

Chords:

That might seem like a lot of info, but we have hardly scratched the surface of what the circle of fifths has to offer. Today we’ll examine three more functions of this diagram. The first: how it shows the chords available in a key. Remember from the episode on the keyboard, a chord is a combination of more than two notes played at the same time. A major chord is made up of a minor third (play audio 1) on top of a major third (audio 2), and a minor chord consists of a major third (audio 3) on top of a minor third (audio 4).

Let’s take C for example. We know that the C major chord is a part of the key of C (audio 5). Look at the two keys on either side of C: F and G. These two chords — F (audio 6) and G (audio 7) — are also major. Now going clockwise around the circle three positions, we find the minor chords in the key of C: D (audio 8), A (audio 9), and E (audio 10). The seventh and last chord in the key of C is the B diminished chord (audio 11), this is made up of two minor thirds on top of each other. So mapping out the chords in the key of C, we find that C is major (audio 5), D is minor (audio 8), E is minor (audio 10), F is major (audio 6), G is major (audio 7), A is minor (audio 9), and B is diminished (audio 11). This pattern of major, minor, minor, major, major, minor, diminished holds true for any major key.

Relative minor keys:

Another function of the circle is that it shows relative minor keys. What I mean by that is: every major has what’s called a relative minor key associated with it. A relative minor has the same key signature as its relative major. I like to think of it as the major key’s shadow. For example, the relative minor of C major is A minor. A minor has the same notes as C major. Here’s the A minor scale (audio 12) and here’s the C major scale (audio 13). Here they are played together (audio 14). The relative minors are written on the inside of the circle in lower case letters.

Greek Modes:

And the last offering of the circle for today has to do with Greek modes. What if we took A major’s key signature and made a scale on D? Well that’s what we call a mode. Modes are when you take one tone’s key signature and put it over another tone. From the circle, I know that A has three sharps: F, C, and G. So let’s play a D scale with these three sharps. The key of D already has F sharp and C sharp, so all we need to do is add G sharp. Listen to what it sounds like (audio 15). This is called the lydian mode, and it’s where you raise the fourth note of a scale. This color of sound sounds unusual and kind of magical. It’s important to note that you can only apply a mode to a tone that’s in the key of the original tone. For example, there’s no C in the key of A major because C is sharp in that key.

Circle of Fifths Activity:

Wow, we learned so much today about physics, math, history, the moon and of course music theory. Now that we’ve established what the circle of fifths is and how it came to be, let’s draw our own to review! Download and print the blank template on our website so you can fill it out yourself. If you can’t do that, you can just grab a piece of paper and something to write with.

Remember, think of the circle as a clock with 12 positions. We start on C at the 12 o’clock position and go up to the fifth note of the C major scale (audio 16) and we arrive at G at 1 o’clock. Then we go from G to the fifth note of that scale which is (Audio 17) at 2 o’clock. We repeat this around the circle and get D, A, E, B, F sharp, D flat, A flat, E flat, B flat, F, and finally we’re back to C.

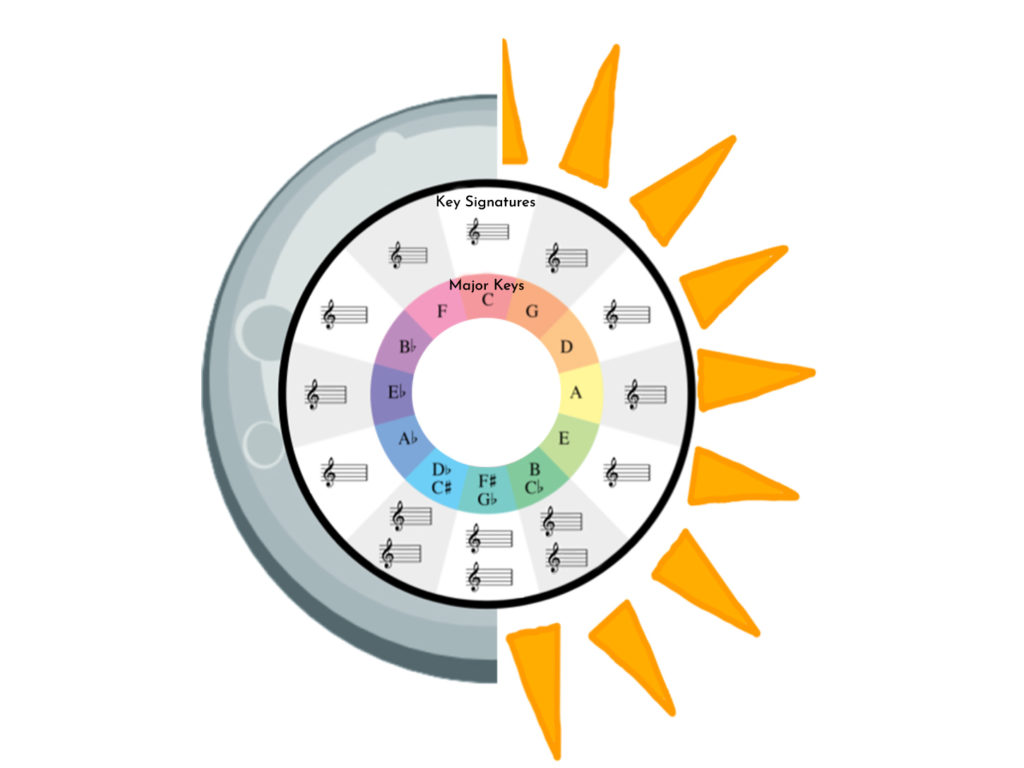
Sharp Key Signatures:

Now let’s draw the key signatures. Let’s start with the sharps, which is the right side of the circle. The number of sharps a key has is the position it is clockwise from C. C has zero sharps, G has one because it is one away from C; D has two, A has three, E has four, B has five, and F sharp has six. To find which notes are sharp in a key signature, we start at F and go clockwise however many positions to the right of C the tone is.

Flat Key Signatures

Now let’s try flats; this is the left side of the circle — or as I like to think of it: the dark side of the moon. The number of flats is how many positions from C the tone is. Which notes are flat is determined by starting on B and counting up the notes towards C.

Relative Minors:

Alright, that’s the first main use of the circle. The next is mapping out the relative minors. Every major key has a relative minor key, which is like its shadow. You draw these on the inside of the circle in lowercase letters. For C major, we have A minor. After A minor, go up a fifth and you get E minor as the relative minor of G major. A fifth up from E is B, then g flat, d flat, a flat, e flat, b flat, f, c, g, d, and finally back to a.

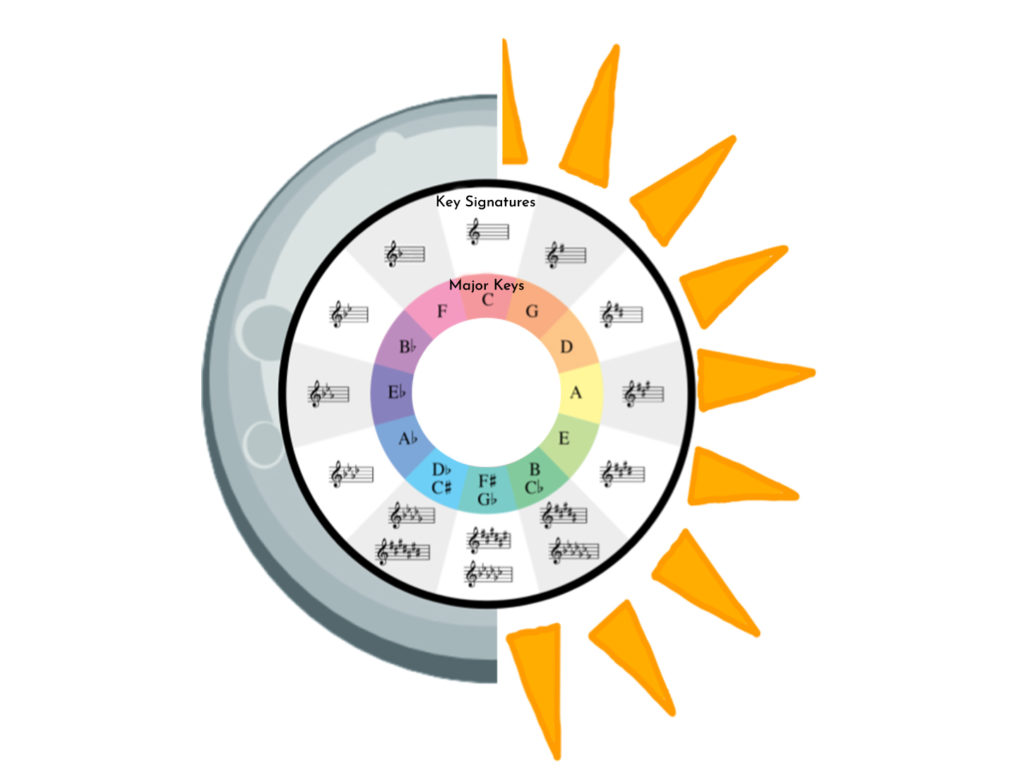
There ya have it! With major keys and key signatures on the outside, and minor keys on the inside, you’ve completed your circle of fifths. Again, this is a treasure map to so many of the hidden secrets of music. Treat it with care. Feel free to decorate it or use it in your future endeavors.

How do non-Western Musicians Play?

An important note to mention is that the circle of fifths and the 12 tone system is popular in Western music, meaning Europe and the Americas. Other cultures have other systems to organize their music, with even more than 12 tones. We call these microtonal systems, where each octave has more than 12 notes. For example, Shruti is a 22 tone system which belongs to Indian classical music. Shruti in Sanskrit means “what is heard,” so the founders of the system decided that you can’t fit in more than 22 unique tones, or shrutis, in an octave. Once you go past 22, the tones sound so similar that you can’t tell them apart. This is a really cool approach to organizing music! Here’s an example of Shruti music:

At the start of this episode, we agreed that we were nearing the finish line of this journey. The finish was still kinda blurry though because we were still a ways away. But now it’s coming into focus. You’re running past the crowds on either side of you towards this finish line, thinking back on all the wonderful things you’ve learned. Through this journey on “The Shape of Music,” you not only tapped into your musical side, but you also learned important lessons in studying cultures from around the world, physics, math, history, and a whole new language. This isn’t the end yet though. Your journeys are far from over. Be on the lookout for a recap episode, where we talk through all of what we learned these past five episodes and put a nice little bowtie on this series.

Check the website for The Shape of Music 5 Part Two!

Remember, this is a two-parter! In the second part of this episode, we will explore so many more uses of the circle of fifths: how it explains our basic chords, why there is a whole new shadow circle of fifths which explores relative minor keys, what Greek modes are and how they’re used, and be sure to check out the podcast version of this episode on the website for more on the circle of fifths—our musical treasure map! Don’t forget that this is a two-parter. Join us in the second half of this episode where we explore how the circle of fifths give us the basic chords in a key, where minor scales fit into this diagram, what Greek modes are, and how a note is not just one note, it comes with a series of overtones found in the circle.

About the author:

Mete Bakircioglu is a senior at Lakeridge High School who strives to empower his generation in his American and international communities through promoting the arts and celebrating cultures. His primary academic interest is political science, and understanding how people can shape society for the betterment of underrepresented communities. As a member of his school’s speech and debate team, Mete loves to study the art of argumentation. Outside of school, he enjoys going on afternoon walks with his dog Lucy and serving on his school district’s school board as the student representative for his high school. Mete also enjoys playing piano and violin. He is currently preparing for OMTA Syllabus evaluation for level 10 piano.